5.1: Number Talk: What's That Exponent?
Solve each equation mentally.
$\frac{100}{1} = 10^x$
$\frac{100}{x} = 10^1$
$\frac{x}{100} = 10^0$
Let’s see what happens when exponents are negative.
Solve each equation mentally.
$\frac{100}{1} = 10^x$
$\frac{100}{x} = 10^1$
$\frac{x}{100} = 10^0$
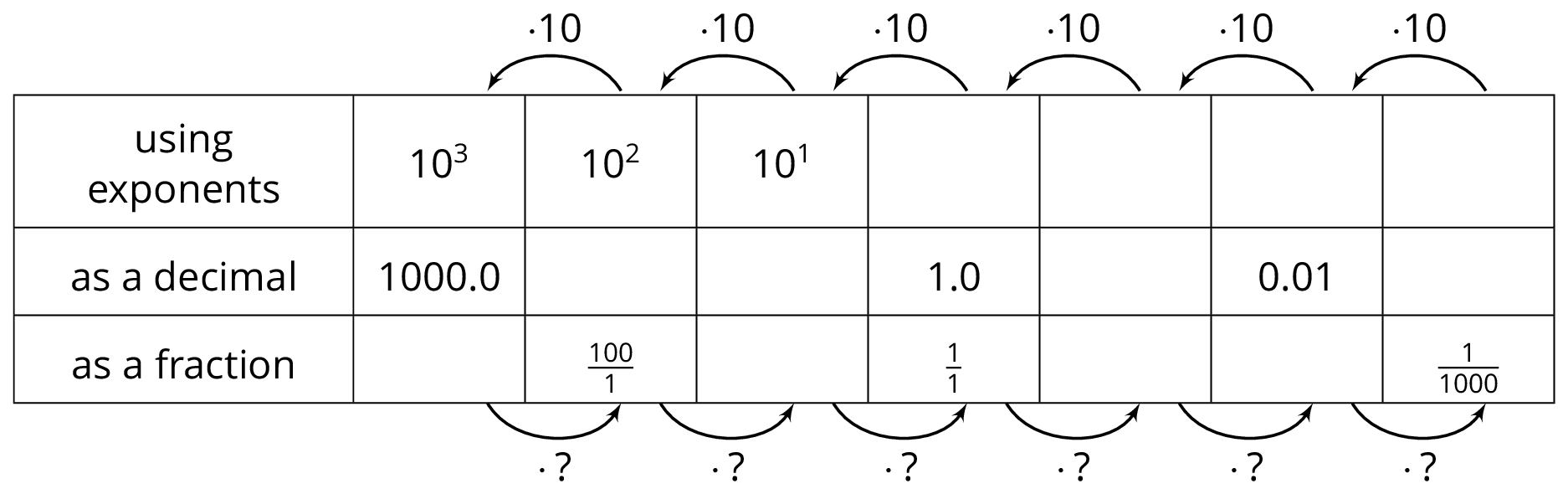
Complete the table to explore what negative exponents mean.
Match the expressions that describe repeated multiplication in the same way:
| Row 1 | $\left(10^2\right)^3$ | $\frac{1}{(10 \boldcdot 10)} \boldcdot \frac{1}{(10 \boldcdot 10)} \boldcdot \frac{1}{(10 \boldcdot 10)}$ |
|---|---|---|
| Row 2 | $\left(10^2\right)^{\text -3}$ | $\left(\frac{1}{10} \boldcdot \frac{1}{10}\right)\left(\frac{1}{10} \boldcdot \frac{1}{10}\right)\left(\frac{1}{10} \boldcdot \frac{1}{10}\right)$ |
| Row 3 | $\left(10^{\text -2}\right)^3$ | $\frac{1}{ \frac{1}{10} \boldcdot \frac{1}{10} }\boldcdot \frac{1}{ \frac{1}{10} \boldcdot \frac{1}{10} } \boldcdot \frac{1}{ \frac{1}{10} \boldcdot \frac{1}{10} }$ |
| Row 4 | $\left(10^{\text -2}\right)^{\text-3}$ | $(10 \boldcdot 10)(10 \boldcdot 10)(10 \boldcdot 10)$ |
Match the expressions that describe repeated multiplication in the same way:
| Row 1 | $\frac{10^2}{10^5}$ | $\frac{ \frac{1}{10} \boldcdot \frac{1}{10} }{ \frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10}\boldcdot \frac{1}{10}\boldcdot \frac{1}{10} }$ |
|---|---|---|
| Row 2 | $\frac{10^2}{10^{\text -5}}$ | $\frac{10 \boldcdot 10}{10 \boldcdot 10 \boldcdot 10 \boldcdot 10 \boldcdot 10}$ |
| Row 3 | $\frac{10^{\text -2}}{10^5}$ | $\frac{ \frac{1}{10} \boldcdot \frac{1}{10} }{ 10 \boldcdot 10\boldcdot 10\boldcdot 10\boldcdot 10 }$ |
| Row 4 | $\frac{10^{\text -2}}{10^{\text -5}}$ | $\frac{ 10 \boldcdot 10 }{ \frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10}\boldcdot \frac{1}{10}\boldcdot \frac{1}{10}}$ |
Match the expressions that describe repeated multiplication in the same way:
| Row 1 | $10^4 \boldcdot 10^3$ | $(10 \boldcdot 10 \boldcdot 10 \boldcdot 10) \boldcdot ( \frac{1}{10} \boldcdot \frac{1}{10}\boldcdot \frac{1}{10})$ |
|---|---|---|
| Row 2 | $10^4 \boldcdot 10^{\text -3}$ | $\left(\frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10}\right) \boldcdot \left( \frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10}\right)$ |
| Row 3 | $10^{\text -4} \boldcdot 10^3$ | $\left(\frac{1}{10}\boldcdot \frac{1}{10} \boldcdot \frac{1}{10} \boldcdot \frac{1}{10}\right) \boldcdot \left(10 \boldcdot 10 \boldcdot 10\right)$ |
| Row 4 | $10^{\text -4} \boldcdot 10^{\text -3}$ | $(10 \boldcdot 10 \boldcdot 10 \boldcdot 10) \boldcdot (10 \boldcdot 10 \boldcdot 10)$ |
Priya, Jada, Han, and Diego are playing a game. They stand in a circle in this order and take turns playing a game.
Priya says, SAFE. Jada, standing to Priya's left, says, OUT and leaves the circle. Han is next: he says, SAFE. Then Diego says, OUT and leaves the circle. At this point, only Priya and Han are left. They continue to alternate. Priya says, SAFE. Han says, OUT and leaves the circle. Priya is the only person left, so she is the winner.
Priya says, “I knew I’d be the only one left, since I went first.”
When we multiply a positive power of 10 by $\frac{1}{10}$, the exponent decreases by 1: $$10^8 \boldcdot \frac{1}{10} = 10^7$$This is true for any positive power of 10. We can reason in a similar way that multiplying by 2 factors that are $\frac{1}{10}$ decreases the exponent by 2: $$\left(\frac{1}{10}\right)^2 \boldcdot 10^8 = 10^6$$
That means we can extend the rules to use negative exponents if we make $10^{\text-2} = \left(\frac{1}{10}\right)^2$. Just as $10^2$ is two factors that are 10, we have that $10^{\text-2}$ is two factors that are $\frac{1}{10}$. More generally, the exponent rules we have developed are true for any integers $n$ and $m$ if we make $$10^{\text-n} = \left(\frac{1}{10}\right)^n = \frac{1}{10^n}$$
Here is an example of extending the rule $\frac{10^n}{10^m} = 10^{n-m}$ to use negative exponents: $$\frac{10^3}{10^5} = 10^{3-5} = 10^{\text-2}$$ To see why, notice that $$\frac{10^3}{10^5} = \frac{10^3}{10^3 \boldcdot 10^2} = \frac{10^3}{10^3} \boldcdot \frac{1}{10^2} = \frac{1}{10^2}$$which is equal to $10^{\text-2}$.
Here is an example of extending the rule $\left(10^m\right)^n = 10^{m \boldcdot n}$ to use negative exponents: $$\left(10^{\text-2}\right)^{3} = 10^{(\text-2)(3)}=10^{\text-6}$$To see why, notice that $10^{\text-2} = \frac{1}{10} \boldcdot \frac{1}{10}$. This means that $$ \left(10^{\text-2}\right)^{3} =\left( \frac{1}{10} \boldcdot \frac{1}{10}\right)^3 = \left(\frac{1}{10} \boldcdot \frac{1}{10}\right) \boldcdot \left( \frac{1}{10} \boldcdot \frac{1}{10}\right)\boldcdot \left(\frac{1}{10}\boldcdot \frac{1}{10}\right) = \frac{1}{10^6} = 10^{\text-6}$$